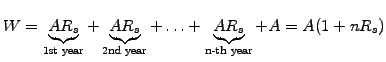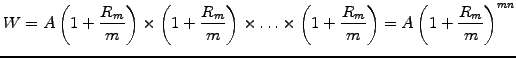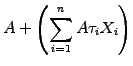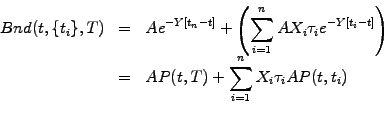Masters: simple, discrete and continuous compounding of interest rates
Consider an amount A invested for an annual interest rate R during n years. If the money earned once a year is not reinvested, the terminal value from a simply compounded calculation leads to
If the money is compounded m times a year and immediately re-invested at the same rate, the terminal value from a discretely compounded calculation becomes
Increasing the compounding frequency to infinity mܥ, the terminal value from the continuously compounded calculation tends to
where the yield Y can be understood as the annual growth rate of the investment. Continuous compounding is often used for simplicity instead of the more realistic discrete compounding; both are in any case simply connected via
where Rm is the discretely and R the continuously compounded annual rate.
For example, take a 1% monthly rate of a unit investment: depending on the compounding, this translates into WAPR=1.01 x 12=1.12, WAPY=1.0112=1.126825, which approaches the continuous annual value of W=exp(0.01 x 12)=1.1275. In other words, the return has an APR of 12% and an APY of 12.68%. Brokerage houses often insure a single certificate of deposit for up to EUR 500,000. The main disadvantage of a certificate of deposit is that it locks up the money for a long time unless a steep penalty is payed... up to half of the return from the interest rate!
A large variety of funds pursue different investment strategies (countries, industries, risk levels, ethical factors): on Feb 23, 2002, the Financial Times newspaper listed more than seven pages with funds... more than shares! In this context, it is good to remember that market indices (such as FTSE-100, Russel 1000, NASDAQ-100) are by definition an arithmetic average; since funds now represent a larger fraction of the market, it is clear that roughly half of the funds under-perform that index. Pursuing a variety of often contradicting strategies can nevertheless be exploited by the marketing departments of the management firms, who simply highlight even few funds that outperformed the index to advertise the skills of all the managers.
Masters: fixed stream of payments of a bond / discount factor.
Throughout its life, a bond generates a predetermined stream of payments
where the amount A is the principal value outstanding at maturity (usually normalized to 1 or 100),
As the name suggests, a zero-coupon bond does not pay any coupon and simply returns the contractual value AP(T,T)=A on the maturity date T. Its present value AP(t,T) measured at time t<T can be calculated from a no-arbitrage argument, provided that the interest rates are fixed and that the issuer is certain to pay the loan back on time. Indeed, investing an equivalent amount of cash on the money market for a yield Y should result in the same final value as when the bond matures; if this were not true and the price lower (alt. higher), it would be possible to buy (alt. sell) bonds in exchange of cash on the money market and generate a risk-free profit at the maturity date. Arbitrageurs would immediately take advantage of such opportunities until the demand (alt. offer) moves the price back to the equilibrium value
In a risk-free economy, the present value of an asset can always be calculated from a price known in the future by multiplication by the discount factor
showing that the present value of a coupon-bearing instrument can always be reduced to a linear combination of zero-coupon bonds (exercise 1.07).
In the real world, the spot price of a bond is determined by the offer and demand from investors and depends also on the credit worthiness of the issuer. Rating agencies such as Standard & Poor, Moody's or KMV use different criteria to judge issuers who are labeled from the safest ``investment grade'' (AAA, AA, A, BBB, of which 2.95% American corporate bonds defaulted in 2002) down to ``speculative'' (BB, B, CCC, CC), ``junk'' or ``default'' (C,D). The price of a bond drops sharply when the risk of defaulting on a debt rises: check the historical value of the Argentinian government bonds as its credit worthiness was finally downgraded from C to D in December 2001.
The spot price quoted for a variety of bonds can be accessed with
a dozen minutes delay free of charge over the Internet (take e.g.
Yahoo,
Bloomberg, etc)
and the closing prices are reported one day later in the press:
for example, on Feb 23, 2002, the
Financial Times
printed the values in 1.3#tab.1.
|
The first row shows a Swedish government bond that matures Jan 2009 and pays a 5% annual coupon: with a good investment grade AA+ and a yield in line with the market's expectations, the price (given as a percentage of the principal value of EUR 1,000) is 0.154% higher than the principal. If you bought this bond on Feb 22, you would now earn 0.03% less than the original coupon.
Marconi's corporate bond expires March 2010 and pays a coupon of 6.375%; after the downgrade of telecom operators and speculations about the company's financial fitness, the coupon is now well below what the market expects for the speculative B+ rating. This explains why the bond lost 65% of its principal value and was now only worth 35.00. If you bought this bond on Feb 22, you could earn a very high yield of 26.73% during the next 10 years, provided that the company does not go bankrupt in the mean time.
Small systematic costs have a large impact on the long term return of a portfolio: investors should never neglect the possibility of tax reductions or outright exemptions when buying municipal, state or government bonds.
In many countries, the tax on dividend income is higher than the tax on the gain in capital - although recent modifications of the taxation in the US may revert this trend. Shareholders therefore prefer to keep the dividend yield low and let the value of shares grow with the company as long as growth remains possible. The valuation of the company's assets, together with the latest results and the expectation of future earnings directly impact on the offer and demand from investors on the stock market (such as NYSE, NASDAQ) which ultimately determines the price of shares.
The spot prices quoted for every share can be read free of charge
on the Internet after only a dozen minutes delay (take e.g.
Yahoo,
Bloomberg,
etc) and the closing prices are reported one day later in the press:
for example, on Feb 23, 2002, the
Financial Times
printed the values in 1.3#tab.2 below.
|
The first row shows that the share from Hilton hotels fell GBP 4.75 to 215.50, in a liquid market with more than 8 million shares exchanged during the trading day. This price is somewhere in the middle of the range over which the share was trading during the last 12 months, as indicated by the high and low ends of the price interval. The price-to-earning ratio shows that it approximatively takes P/E=11.9 years for the company earnings to add up to the original purchase price (``paying back your investment'') if the earnings remain fixed. Assuming a small one percent growth G=0.01 for a mature industry, you can show (exercise 1.06) that this corresponds to an expected return on investment of G+E/P=0.01 +1/11.9=0.094 or 9.4%, which is indeed much larger than the 4% dividend yield payed in cash to the shareholders; investors should therefore expect Hilton's share price to rise by an annual 5.4%.
AstraZenca pharmaceuticals have been growing very fast during the last years and, expecting that this will continue into the future, investors are willing to pay a much larger price-to-earning ratio of 29.9. For the sake of simplicity, assume that the exponential growth reached from the Low to the quoted Price during exactly one year, so that the growth rate can be estimated from G=ln(P2/P1)/(t2-t1)=ln(3519/2724)=0.25. This translates into astronomical returns G+E/P=0.25 +0.033=0.28 which exceed by far the 3.3% justified by the earnings and the 1.4% payed as dividends.
Finally, note the near collapse of Marconi's share from GBP 800 to 16.75, which shows that the company has large financial difficulties and may go bankrupt, i.e. the share value drop to zero forever. This is consistent with the low credit worthiness perceived for its corporate bond (1.3#tab.1) and underlines the fact that a high investment risk can lead to large losses.


 SYLLABUS Previous: 1.2 Capital and markets
Up: 1 INTRODUCTION
Next: 1.4 Modern portfolio theory
SYLLABUS Previous: 1.2 Capital and markets
Up: 1 INTRODUCTION
Next: 1.4 Modern portfolio theory