2.4 Hedging parameters, portfolio sensitivity

SYLLABUS Previous: 2.3 Convertible bonds Up: 2 A VARIETY OF Next: 2.5 Computer quiz

2.4 Hedging parameters, portfolio sensitivity $\diamondsuit$

[ SLIDE delta - gamma - theta - vega - rho || same VIDEO as previous section: modem - LAN - DSL ]

The put-call parity in (2.1.3#eq.2) shows how a particular combination of options with the underlying can be used to exactly cancel the investment risk in a very simple situation. The same trick could in principle be used for all the securities in a portfolio

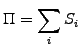$\displaystyle \Pi=\sum_i S_i$

(2.4#eq.1)

Such a hedging strategy is however neither practical nor does it in general produce the desired effect: remember that, to achieve a certain return, investors need to take a limited amount of risk - albeit in a controlled manner that can be monitored to make sure that in the long term the investment survives even large market fluctuations. To quantify the sensitivity to small changes of a limited number of parameters, the portfolio value is often expanded into a Taylor series and the dominant terms labelled using Greek letters.
The largest contribution is usually delta and measures how the value of the portfolio changes with the value of each individual asset

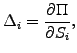$\displaystyle \Delta_i=\frac{\partial \Pi}{\partial S_i},$

(2.4#eq.2)

Gamma quantifies smaller effects due to the curvature

$\displaystyle \Gamma_i=\frac{\partial^2 \Pi}{\partial S_i^2}$

(2.4#eq.3)

and vega (not a Greek letter) measures the sensitivity to changes in the volatility

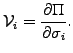$\displaystyle \mathcal{V}_i=\frac{\partial \Pi}{\partial \sigma_i}.$

(2.4#eq.4)

An extension including variation from any subset of the assets is straightforward using gradients in multiple dimensions. The so-called theta measures the decay of the value with time

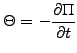$\displaystyle \Theta=-\frac{\partial \Pi}{\partial t}$

(2.4#eq.5)

and rho the sensitivity to small variations in the interest rate

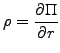$\displaystyle \rho=\frac{\partial \Pi}{\partial r}$

(2.4#eq.6)

Finally, when a bond paying coupons A_i at times t_i is discounted to the present value B=å A_i exp(-r t_i) at a yield Y, the duration measures how long on average the investor has to wait before he recieves cash payments

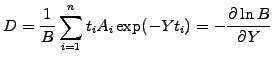$\displaystyle D=\frac{1}{B}\sum_{i=1}^n t_i A_i\exp(-Y t_i) = - \frac{\partial\ln B}{\partial Y}$

(2.4#eq.7)

By monitoring and limiting the dependences to some of these factors, hedgers can at least start to identify and reduce if not eliminate the short term risk in a portfolio.

SYLLABUS Previous: 2.3 Convertible bonds Up: 2 A VARIETY OF Next: 2.5 Computer quiz